On the Road Again According to Exercise 4 the Probability

Probability means possibility. It is a branch of mathematics that deals with the occurrence of a random event. The value is expressed from cypher to one. Probability has been introduced in Maths to predict how likely events are to happen. The meaning of probability is basically the extent to which something is likely to happen. This is the basic probability theory, which is also used in the probability distribution, where you will learn the possibility of outcomes for a random experiment. To find the probability of a single event to occur, offset, we should know the full number of possible outcomes.

Learn More here: Report Mathematics

Probability Definition in Math

Probability is a measure of the likelihood of an event to occur. Many events cannot be predicted with total certainty. Nosotros can predict just the chance of an event to occur i.eastward. how likely they are to happen, using information technology. Probability tin range in from 0 to i, where 0 means the event to exist an impossible i and ane indicates a certain event. Probability for Class 10 is an of import topic for the students which explains all the basic concepts of this topic. The probability of all the events in a sample space adds up to one.

For instance, when nosotros toss a coin, either we get Head OR Tail, only two possible outcomes are possible (H, T). Simply if we toss 2 coins in the air, in that location could exist three possibilities of events to occur, such equally both the coins evidence heads or both show tails or one shows heads and ane tail, i.e.(H, H), (H, T),(T, T).

Download this lesson as PDF: – Download PDF Here

Formula for Probability

The probability formula is defined as the possibility of an event to happen is equal to the ratio of the number of favourable outcomes and the total number of outcomes.

Probability of event to happen P(E) = Number of favourable outcomes/Total Number of outcomes

Sometimes students go mistaken for "favourable consequence" with "desirable outcome". This is the basic formula. But there are some more formulas for unlike situations or events.

Solved Examples

1) In that location are 6 pillows in a bed, three are red, 2 are yellow and 1 is blue. What is the probability of picking a yellowish pillow?

Ans: The probability is equal to the number of xanthous pillows in the bed divided past the total number of pillows, i.eastward. 2/6 = i/three.

two) In that location is a container total of coloured bottles, cerise, blue, green and orange. Some of the bottles are picked out and displaced. Sumit did this k times and got the following results:

• No. of bluish bottles picked out: 300
• No. of red bottles: 200
• No. of light-green bottles: 450
• No. of orangish bottles: 50

a) What is the probability that Sumit will pick a green canteen?

Ans: For every 1000 bottles picked out, 450 are green.

Therefore, P(green) = 450/one thousand = 0.45

b) If at that place are 100 bottles in the container, how many of them are probable to exist green?

Ans: The experiment implies that 450 out of thou bottles are green.

Therefore, out of 100 bottles, 45 are dark-green.

Probability Tree

The tree diagram helps to organize and visualize the unlike possible outcomes. Branches and ends of the tree are two main positions. Probability of each branch is written on the branch, whereas the ends are containing the final event. Tree diagrams are used to figure out when to multiply and when to add. You can run across beneath a tree diagram for the coin:

Types of Probability

At that place are iii major types of probabilities:

• Theoretical Probability
• Experimental Probability
• Axiomatic Probability

Theoretical Probability

It is based on the possible chances of something to happen. The theoretical probability is mainly based on the reasoning behind probability. For example, if a money is tossed, the theoretical probability of getting a head will exist ½.

Experimental Probability

It is based on the basis of the observations of an experiment. The experimental probability can be calculated based on the number of possible outcomes by the total number of trials. For instance, if a coin is tossed x times and heads is recorded six times then, the experimental probability for heads is vi/10 or, 3/five.

Axiomatic Probability

In axiomatic probability, a set up of rules or axioms are set which applies to all types. These axioms are prepare past Kolmogorov and are known as Kolmogorov's iii axioms. With the axiomatic arroyo to probability, the chances of occurrence or non-occurrence of the events tin can be quantified. The evident probability lesson covers this concept in detail with Kolmogorov's three rules (axioms) along with various examples.

Conditional Probability is the likelihood of an event or outcome occurring based on the occurrence of a previous event or consequence.

Probability of an Outcome

Presume an result E tin occur in r ways out of a sum of n probable or possible every bit likely ways. Then the probability of happening of the event or its success  is expressed every bit;

P(E) = r/n

The probability that the effect will non occur or known as its failure is expressed as:

P(Eastward') = (n-r)/north = 1-(r/n)

Due east' represents that the event will not occur.

Therefore, now we can say;

P(Eastward) + P(East') = one

This means that the total of all the probabilities in whatsoever random test or experiment is equal to 1.

What are Equally Likely Events?

When the events take the same theoretical probability of happening, then they are chosen equally probable events. The results of a sample infinite are chosen every bit likely if all of them accept the aforementioned probability of occurring. For instance, if you throw a die, so the probability of getting 1 is i/6. Similarly, the probability of getting all the numbers from 2,3,four,five and six, one at a time is 1/six. Hence, the following are some examples of equally probable events when throwing a die:

• Getting 3 and 5 on throwing a die
• Getting an even number and an odd number on a die
• Getting 1, 2 or 3 on rolling a die

are equally likely events, since the probabilities of each outcome are equal.

Complementary Events

The possibility that in that location will be only two outcomes which states that an event will occur or non. Similar a person will come or not come to your firm, getting a task or not getting a job, etc. are examples of complementary events. Basically, the complement of an event occurring in the verbal opposite that the probability of information technology is not occurring. Some more than examples are:

• It will rain or non rain today
• The student will pass the test or not pass.
• Yous win the lottery or you don't.

Too, read:

• Independent Events
• Mutually Exclusive Events

Probability Theory

Probability theory had its root in the 16th century when J. Cardan, an Italian mathematician and doctor, addressed the first work on the topic, The Volume on Games of Chance. Afterwards its inception, the knowledge of probability has brought to the attention of corking mathematicians. Thus, Probability theory is the branch of mathematics that deals with the possibility of the happening of events. Although there are many singled-out probability interpretations, probability theory interprets the concept precisely by expressing it through a set of axioms or hypotheses. These hypotheses help form the probability in terms of a possibility space, which allows a mensurate holding values between 0 and 1. This is known equally the probability measure, to a prepare of possible outcomes of the sample infinite.

Probability Density Office

The Probability Density Office (PDF) is the probability function which is represented for the density of a continuous random variable lying between a certain range of values. Probability Density Office explains the normal distribution and how mean and deviation exists. The standard normal distribution is used to create a database or statistics, which are frequently used in scientific discipline to represent the real-valued variables, whose distribution is not known.

Probability Terms and Definition

Some of the important probability terms are discussed here:

Term	Definition	Example
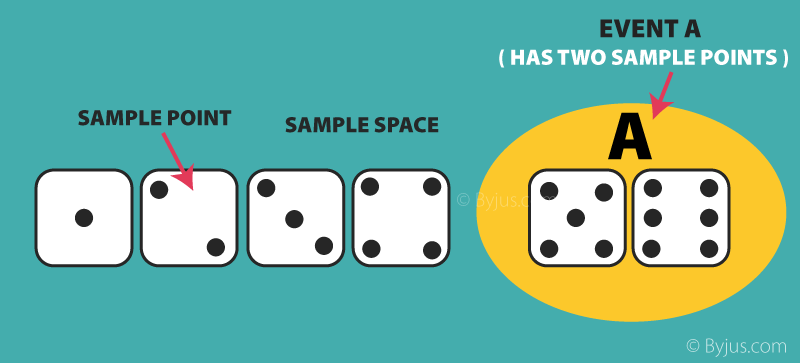
Sample Space	The ready of all the possible outcomes to occur in any trial	Tossing a coin, Sample Space (South) = {H,T} Rolling a die, Sample Infinite (S) = {ane,2,3,four,5,6}
Sample Indicate	It is ane of the possible results	In a deck of Cards: four of hearts is a sample point. The queen of clubs is a sample bespeak.
Experiment or Trial	A serial of deportment where the outcomes are always uncertain.	The tossing of a coin, Selecting a menu from a deck of cards, throwing a dice.
Issue	It is a single outcome of an experiment.	Getting a Heads while tossing a coin is an event.
Outcome	Possible outcome of a trial/experiment	T (tail) is a possible consequence when a coin is tossed.
Costless event	The not-happening events. The complement of an event A is the upshot, not A (or A')	Standard 52-card deck, A = Depict a heart, and so A' = Don't draw a heart
Impossible Event	The event cannot happen	In tossing a coin, impossible to get both head and tail at the same time

Applications of Probability

Probability has a broad variety of applications in real life. Some of the common applications which we see in our everyday life while checking the results of the post-obit events:

• Choosing a menu from the deck of cards
• Flipping a coin
• Throwing a die in the air
• Pulling a ruby-red ball out of a bucket of red and white assurance
• Winning a lucky draw

Other Major Applications of Probability

• It is used for risk assessment and modelling in various industries
• Weather forecasting or prediction of conditions changes
• Probability of a team winning in a sport based on players and force of team
• In the share marketplace, chances of getting the hike of share prices

Problems and Solutions on Probability

Question one: Find the probability of 'getting three on rolling a die'.

Solution:

Sample Space = Southward = {1, 2, three, 4, five, half dozen}

Total number of outcomes = n(S) = 6

Allow A exist the upshot of getting iii.

Number of favourable outcomes = n(A) = i

i.e. A  = {3}

Probability, P(A) = n(A)/northward(S) = 1/half dozen

Hence, P(getting iii on rolling a die) = 1/6

Question ii: Draw a random card from a pack of cards. What is the probability that the card fatigued is a face menu?

Solution:

A standard deck has 52 cards.

Total number of outcomes = due north(Due south) = 52

Allow E be the event of cartoon a confront card.

Number of favourable events = n(E) = four 10 three = 12 (considered Jack, Queen and King only)

Probability, P = Number of Favourable Outcomes/Total Number of Outcomes

P(Due east) = n(Due east)/n(S)

= 12/52

= iii/13

P(the card drawn is a confront carte du jour) = 3/xiii

Question 3: A vessel contains 4 blue balls, 5 ruby balls and eleven white balls. If three balls are fatigued from the vessel at random, what is the probability that the first ball is ruby, the 2nd ball is blueish, and the third brawl is white?

Solution:

Given,

The probability to become the offset brawl is red or the first consequence is 5/twenty.

Since nosotros have drawn a ball for the commencement event to occur, then the number of possibilities left for the 2nd event to occur is 20 – one = 19.

Hence, the probability of getting the 2d ball as blue or the second event is four/19.

Again with the showtime and 2nd event occurring, the number of possibilities left for the third upshot to occur is 19 – ane = 18.

And the probability of the third ball is white or the tertiary issue is 11/xviii.

Therefore, the probability is 5/20 x 4/xix x 11/18 = 44/1368 = 0.032.

Or we tin can express information technology every bit: P = 3.two%.

Question 4: Two dice are rolled, detect the probability that the sum is:

1. equal to 1
2. equal to 4
3. less than 13

Solution:

To find the probability that the sum is equal to 1 we accept to starting time determine the sample space S of ii dice as shown beneath.

Due south = { (ane,1),(1,2),(ane,3),(one,4),(1,five),(1,6)

(2,one),(2,2),(2,3),(2,four),(2,5),(2,6)

(3,ane),(3,2),(iii,three),(3,iv),(iii,v),(3,6)

(4,one),(4,two),(4,iii),(4,4),(four,v),(4,6)

(five,i),(5,2),(v,3),(five,four),(v,five),(v,6)

(6,1),(6,ii),(6,three),(half-dozen,4),(6,5),(6,6) }

So, n(Due south) = 36

1) Let E be the event "sum equal to 1". Since, there are no outcomes which where a sum is equal to 1, hence,

P(E) = due north(E) / n(S) = 0 / 36 = 0

two) Let A exist the event of getting the sum of numbers on dice equal to 4.

Three possible outcomes give a sum equal to 4 they are:

A = {(1,iii),(2,2),(iii,1)}

northward(A) = 3

Hence, P(A) = n(A) / northward(S) = three / 36 = one / 12

3) Allow B exist the event of getting the sum of numbers on dice is less than 13.

From the sample space, we can see all possible outcomes for the event B, which gives a sum less than B. Like:

(1,one) or (one,6) or (2,6) or (6,6).

So you lot tin see the limit of an effect to occur is when both dies accept number 6, i.east. (6,6).

Thus, n(B) = 36

Hence,

P(B) = n(B) / n(S) = 36 / 36 = one

Video Lectures

Introduction

Solving Probability Questions

Probability Important Topics

Probability Important Questions

Probability Problems

1. Two dice are thrown together. Observe the probability that the production of the numbers on the acme of the dice is:
   (i) 6 (ii) 12 (iii) vii
2. A bag contains 10 ruddy, 5 blue and 7 green assurance. A brawl is drawn at random. Find the probability of this brawl being a
   (i) cerise ball (ii) green ball (iii) not a blueish ball
3. All the jacks, queens and kings are removed from a deck of 52 playing cards. The remaining cards are well shuffled then one card is drawn at random. Giving ace a value ane similar value for other cards, find the probability that the carte du jour has a value
   (i) 7 (ii) greater than vii (3) less than 7
4. A dice has its 6 faces marked 0, i, 1, 1, six, half-dozen. 2 such dice are thrown together and the total score is recorded.
   (i) How many unlike scores are possible?
   (ii) What is the probability of getting a full of 7?

Ofttimes Asked Questions on Probability

What is probability? Give an case

Probability is a branch of mathematics that deals with the occurrence of a random event. For example, when a coin is tossed in the air, the possible outcomes are Head and Tail.

What is the formula of probability?

The probability formula is defined as the possibility of an event to happen is equal to the ratio of the number of outcomes and the total number of outcomes.

What are the different types of probability?

At that place are 3 major types of probabilities:
Theoretical Probability
Experimental Probability
Axiomatic Probability

What are the bones rules of probability?

If A and B are two events, then;
P ( A ∪ B ) = P ( A ) + P ( B ) − P ( A ∩ B )
P ( A ∩ B ) = P ( B ) ⋅ P ( A | B )

mcdonaldaromided.blogspot.com

Source: https://byjus.com/maths/probability/

Share this post